
Sound energy is decreased by a factor of 100 Sound energy is increased by a factor of 100 Sound energy is decreased by a factor of 10 Sound energy is increased by a factor of 10
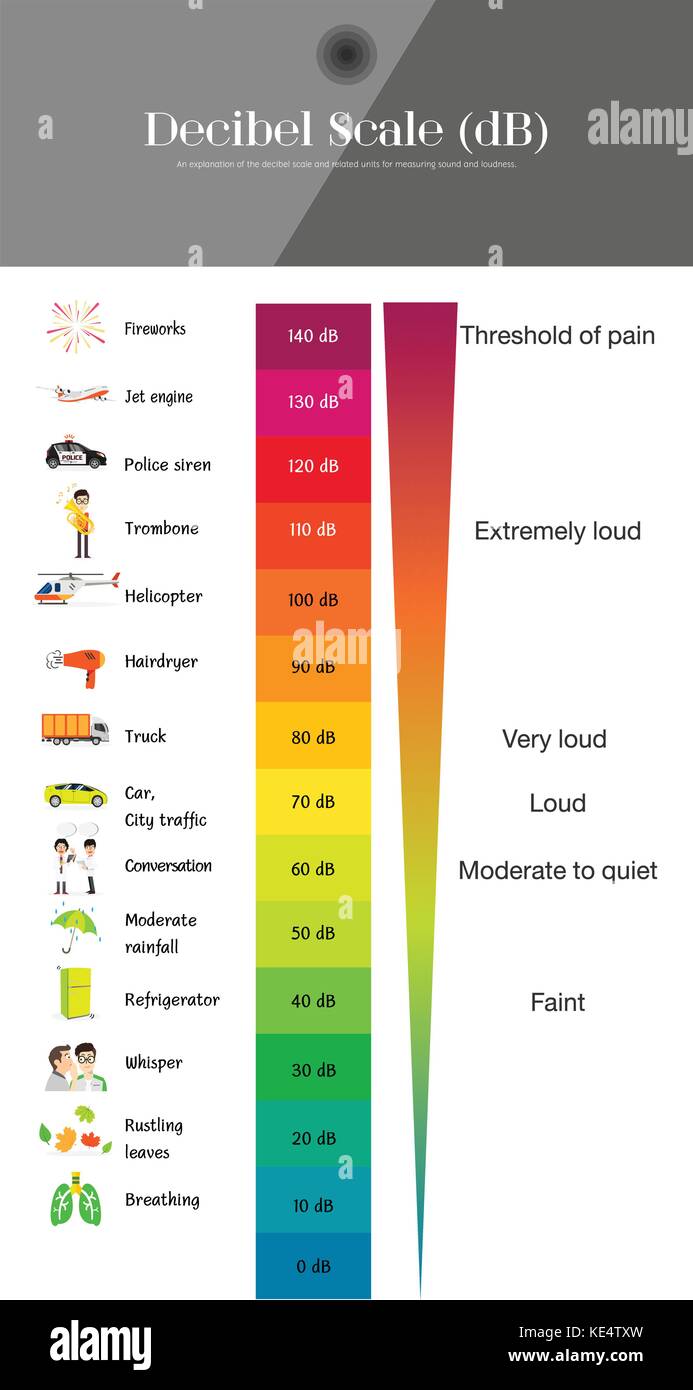
The table below summarises this: The basic rules for working with decibels Change in dB The use of the dB unit makes it easier to work out workplace noise level changes if we use these rules. It is based on orders of magnitude, rather than a standard linear scale, so each mark on the decibel scale is the previous mark multiplied by a value. This scale in this instance is the decibel scale.Ī logarithmic scale is used when there is a large range of quantities. So, to express levels of sound meaningfully in numbers that are more manageable, a logarithmic scale is used, using 10 as the base, rather than a linear one. It would be tricky to keep an eye on those 14 digits varying in front of you. If you think about it, it would be very difficult to manufacture a sound level meter that had a linear performance, especially bearing in the mind the range of noise sources to be measured in a work environment. A noise meter uses a display with a range and resolution to approximate to the ear’s dynamic range, usually the upper range rather than the quiet part. When you measure noise levels with a noise meter, you measure the intensity of noise in units called decibels, expressed as dB(A). “Every 3dB change represents a doubling or halving of sound energy” 3dB rule when measuring noise at work This 3dB rule is important to remember when you come to measure noise as well as analyse and predict individual exposure. If the pin dropping has a sound level of 10dB (decibels) then two pins would have a level of 13dB. In a work context, this means that a small increase in the number of decibels results in a huge change in the amount of noise and, as such, the potential to cause damage to your hearing. When this sound is doubled this is equated to a rise of 3dB (decibels), using a logarithmic scale*. It can, however, distinguish the significant multiplying of the energy of the sound. 3dB rule and the doubling of sound energyĪlthough the human ear can distinguish the rise in level between one or two pins dropping, it cannot distinguish between 10,000,000,000,000 pins and 10,000,000,000,001 pins or even 10,100,000,000,000 because it is not a linear device.

This is something that we cover at length during our noise awareness training courses with our safety professionals. It would take 10,000,000,000,000 pins dropping simultaneously to measure the same sound level as the jet engine. Therefore it can hear the sound of a pin dropping close-by as well as the roar of a jet engine. It has a mechanism that reduces its sensitivity as the sound level rises and it also has the remarkable ability to handle an enormous range of sound levels. We say this often in this blog but, truly, the ear is an extremely versatile and an amazing hearing device. Here, we have tried to explain in simple terms how it works. At first, the 3dB rule can appear a challenging concept but, once you are used to it, it should cause you little difficulty. This is because changes in noise levels can have a significant impact on how you manage it and keep within the legal limits of exposure. If your work involves controlling noise in the workplace, especially if you are responsible for monitoring noise exposure levels, you will need to understand the 3dB rule.
DECIBEL LOGARITHMIC SCALE HOW TO
What is the 3dB rule and how to understand it?


 0 kommentar(er)
0 kommentar(er)
